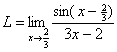Honors AP Calculus / Mr. Hansen	Name: _______________________________
9/24/2009	READ INSTRUCTIONS IN EACH PART! ______

Test #1 (100 points): Calculator permitted throughout

	Part I: Multiple Choice (0 points off for a correct answer, –5 for an error, –4 for an omission). Write the capital letter of the best choice in the blank provided. There is no partial credit, and work is ignored. Do not circle or black out anything. Points may be deducted if you do not follow instructions.
1. ___	For our purposes in HappyCal, a definition is . . .
	(A) an explanation of a term	(D) the contrapositive of a theorem
	(B) a conditional statement	(E) a formal system
	(C) a biconditional (“iff”) statement
2. ___	In the formal definition of , what is the reason for using a punctured -neighborhood of c in the clause that says 0 < | x – c | < must imply | f (x) – L | < , instead of simply basing the definition on an open interval where x (c – , c + )?
	(A) An open interval is not a neighborhood of a point.
	(B) There is no good reason; an open interval would work just as well as a punctured -neighborhood, but there is a tradition in calculus textbooks of using both and in the definition of limit.
	(C) The question is posed incorrectly. The formal definition of limit requires the function values to lie within a punctured -neighborhood of L, i.e., the set of y values such that 0 < | y – L | < , where y = f (x).
	(D) The question is posed incorrectly. The formal definition of limit requires the function values to lie within a punctured -neighborhood of L, i.e., the set of x values such that 0 < | x – L | < , where y = f (x).
	(E) We wish our definition to make no requirement that f (c) exists.
3. ___	Which of the following is true regarding the formal system we call “the calculus”?
	(A) It is never appropriate to have “jackets off” when studying the calculus.
	(B) Deep understanding and months of instruction are required in order to compute numeric answers.
	(C) Computers can obtain correct numeric answers to many problems.
	(D) The calculus is (mostly) a waste of time, since all important applications have now been formalized.
	(E) The two most important application areas rely exclusively upon the differential calculus, not the integral calculus.
4. ___	An equation containing a derivative (of some order) is called a . . .
	(A) derivative	(D) differential equation (“diff. eq.”)
	(B) derivative equation	(E) definite integral
	(C) differential
	Part II: Short Answer (6 points each, with essentially no partial credit).
	No work is required or expected for #5 through #10. All numeric answers must be either exact or rounded to 3 decimal places. Be sure to hit MODE to place your calculator in radian mode.
5.	Let . Conjecture (do not prove) the value of L. Answer: L = ____
6.	Fill in the blanks: The calculus was developed in the 17th century by ______________ (name at least one of the co-developers). “A calculus” refers to any ______________ system, by which we mean a system of symbol manipulation.
7.	State the formal definition of for an arbitrary function f. You may use words, symbols, or a mixture.
	______________________________________________________________________________
	______________________________________________________________________________
8.	Use any method (MATH 8, graphing with 2nd CALC 6, etc.) to find if g(x) = 3x^2 – 3. Answer: = ____
9.	Fill in the blanks: The symbol that means the same as
	:
	|
	or “s.t.”
	in mathematics is _______________ . The four-letter abbreviation that typically can be used to begin a proof by contradiction (a.k.a. indirect proof) is ________________ .
10.	Mathematicians do not always begin a “” proof with the abbreviation wlog. For example, they may write, “Take ” when they really mean, “Wlog, take .” Nevertheless, the “spirit of wlog” is important in most proofs. Briefly explain why.
	___________________________________________________________________________
	___________________________________________________________________________
	Part III: Longer Answer (16 points each, partial credit available).
	Show your work. Be neat and clear. The numeric answer, if any, is worth much less than the justification you provide. Complete sentences are not required.
11.	The right-hand derivative of function f at point c is defined as follows: . If f (c) is undefined, then the right-hand derivative of f at c is also undefined.
(a)	Estimate the right-hand derivative of f (x) = x^x at the point c = 0. Note that although 0^0 is normally taken to be undefined, we will stipulate that f (0) = 1. Use your calculator, and share your computations.
(b)	Explain briefly why is not the same concept as the right-hand derivative at c.
12.	Give examples (using either an explicit definition or, more helpfully, a sketch) of functions f, g, and h such that
(a)	the right-hand derivative of f at point c equals
(b)	the right-hand derivative of g at point c does not exist, but does exist as a real number
(c)	neither the right-hand derivative of h at point c nor exists
13.	If we compute the definite integral of a velocity function (miles per hour) with respect to time (hours), what units will our answer be in? _______________ What does the answer mean in a real-world sense? ______________________________________________________________________________ ______________________________________________________________________________
	What gauge on a car displays the derivative (instantaneous rate of change) of the car’s displacement function? ___________________________
	I have, in my car, an instantaneous mileage display that tells me, at any moment, the approximate fuel economy (in miles per gallon) that my car is achieving. In other words, this display is much like a speedometer, except that instead of telling me my instantaneous forward velocity in mph, it tells me my instantaneous fuel economy in mpg. If I am going downhill on the GW Parkway in Virginia, I can sometimes hit 80 or 90 mpg, but when I drive around in the Cathedral garage, I am lucky to achieve 5 mpg. An interesting thing to do might be to plot all these mpg readings (measured, say, every 0.1 mile) to make a graph. Since the mpg data represent instantaneous rates of change in my displacement per unit of fuel, these readings are (circle one) derivatives   average rates of change   definite integrals. Now, just as I can take a definite integral of the car’s velocity function with respect to time, I might want to take a definite integral of the car’s mpg function with respect to distance as a way of determining my total fuel consumption. Since my readings are made only every 0.1 mile, my graph might well have some bumps and spikes in it, but I could certainly use the trapezoid rule or some comparable technique to estimate the definite integral. However, this does not work for computing total fuel consumption. Why not? (Hint: Think of the units.) ______________________________________________________________________________ ______________________________________________________________________________ What function could I integrate with respect to distance as a way of determining the total amount of fuel consumed? (Hint: There is no gauge on my car that displays this function, at least not directly, but if I am ambitious enough to record data readings every 0.1 mile, I could certainly figure out a way to compute this function.) ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________