|
Geometry / Mr. Hansen |
Name: _________________________ |
Test
on Chapter 5 (Short Answer Portion)
|
|
Short answer (5 points each). No partial credit in
most cases. |
|
|
|
|
1. |
Given: Distinct lines l, m, n with l || m, and n intersecting both of the other two. |
|
|
|
|
|
Make a sketch. Label the
lines correctly (including tick marks), and label the 8 angles that are
formed. Raise your hand so that I know that everyone’s numbers are
compatible. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
2. |
List all pairs of angles
that are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
3. |
Suppose in #1 that it is
not known that l || m. List all pairs of angles which, if known
to be supplementary, would be sufficient to prove that l || m. |
|
Page 2 (E period version) |
|
|
|
|
|
|
Identify each quadrilateral without “overreaching.” |
|
|
|
|
4. |
All 4 sides are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
5. |
There are two consecutive
angles that are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
6. |
Diagonals bisect the
quadrilateral’s angles. |
|
|
|
|
|
|
|
|
|
|
|
|
|
7. |
There is a diagonal that is
the ^ bisector of the other. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Always, Sometimes, Never. |
|
|
|
|
8.___ |
A square is a kite. |
|
|
|
|
|
|
|
|
|
|
|
|
|
9.___ |
An isosceles trapezoid is a
rectangle. |
|
|
|
|
|
|
|
|
|
|
|
|
|
10.___ |
A parallelogram has
congruent diagonals. |
|
Page 2 (version for most F period students) |
|
|
|
|
|
|
Identify each quadrilateral without “overreaching.” |
|
|
|
|
4. |
All 4 angles are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
5. |
There are two consecutive sides
that are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
6. |
Diagonals are congruent. |
|
|
|
|
|
|
|
|
|
|
|
|
|
7. |
Each diagonal is the ^ bisector of the other. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Always, Sometimes, Never. |
|
|
|
|
8.___ |
A square is a kite. |
|
|
|
|
|
|
|
|
|
|
|
|
|
9.___ |
A rhombus is a rectangle. |
|
|
|
|
|
|
|
|
|
|
|
|
|
10.___ |
A trapezoid has diagonals
that bisect all the angles of the quadrilateral. |
|
Geometry / Mr. Hansen |
Name: _________________________ |
Test
on Chapter 5 (Free Response/Computation)
5 pts. each for
#6-13
|
6. |
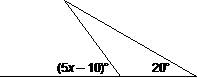
Find the restrictions on x. |
|
|
|
|
|
|
7. |
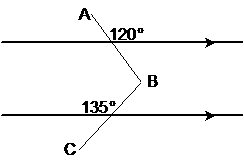
Find mŠB. |
|
|
|
|
|
|
8. |
In this problem, you were given trapezoid EASY with segment EY and segment AS as bases. Given mŠA = 4x, mŠY = x + 60, and AS = x – 3, find AS. [Note: In the problem as originally written, the bars over EY and AS were correct, but the bar over AS was a typographical error since AS is a length, not a segment.] |
|
|
|
|
|
|
9. |
Given: rhombus AHDR with
diagonals intersecting at Y, perimeter = 52, and mŠHAR = 60 |
|
|
|
|
|
|
10. |
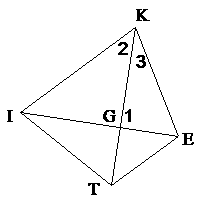
Given: Kite KITE, mŠ1 = 6x, mŠ2 = x + 20 |
|
|
|
|
|
|
|
For problems 11 and 12, you were given a rhombus, with vertices T(3, 2), O(8, 2), P(11, 6), and S on the same y value as P. You were also given that the diagonals intersect at V. |
|
|
|
|
|
|
11. |
Find the coordinates of V. |
|
|
|
|
|
|
12. |
Find the slope of segment
SO. |
|
|
|
|
|
|
13. |
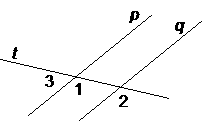
Given: p || q, mŠ1 = 2x + 20, mŠ2 = 3x – 50 |
|
|
|
|
|
|
PROOF |
You were given scalene DPIG, with segment PT being the altitude to segment IG, and you were asked to provide an indirect proof (a.k.a. proof by contradiction) to show that segment PT could not be the median to segment IG. |
|